Distinguishing modulated oscillations from coloured noise in multivariate datasets. Climate Dynamics.
Abstract
Extended empirical orthogonal functions (EEOFS), alternatively known as multi-
channel singular systems (or singular spectrum) analysis (MSSA), provide
a natural method of extracting oscillatory modes of variability from
multivariate data. The eigenfunctions of some simple non-oscillatory
noise processes are, however, also solutions to the wave equation, so the
occurrence of stable, wave-like patterns in EEOF/MSSA is not sufficient
grounds for concluding that the data exhibits oscillations. We present a
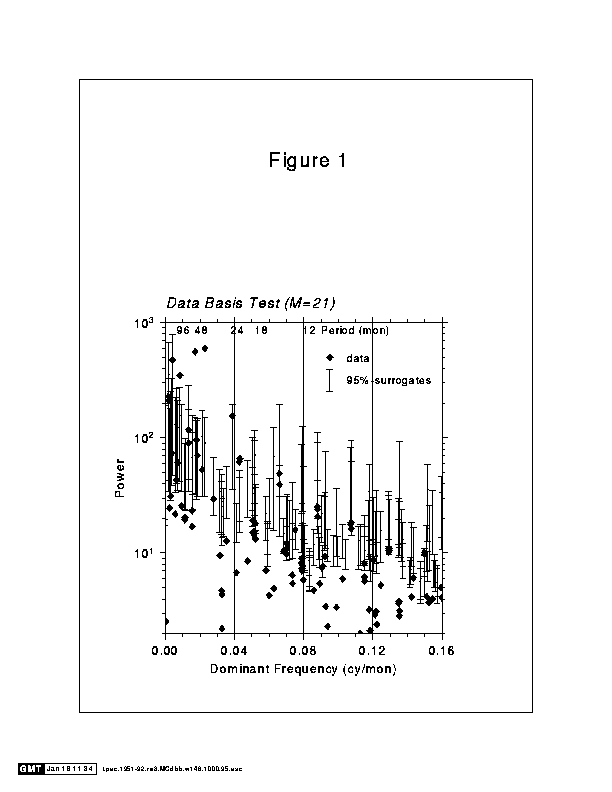
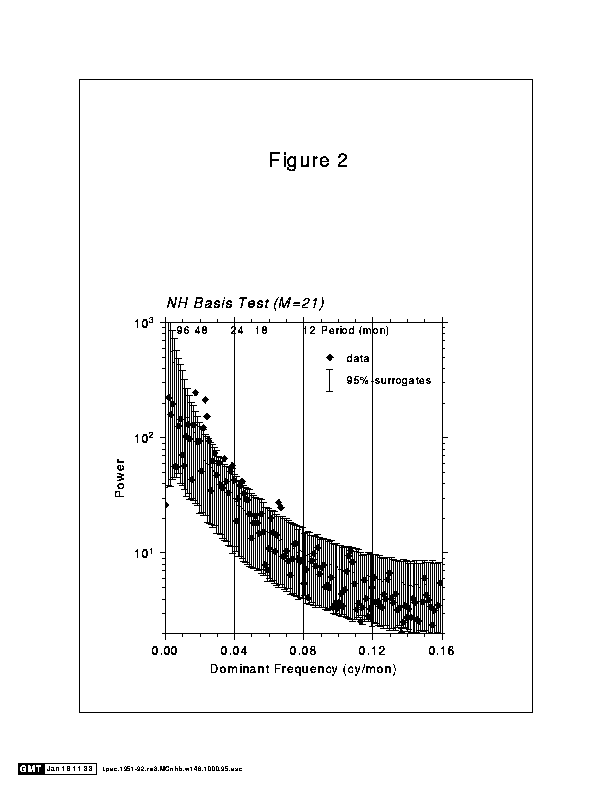
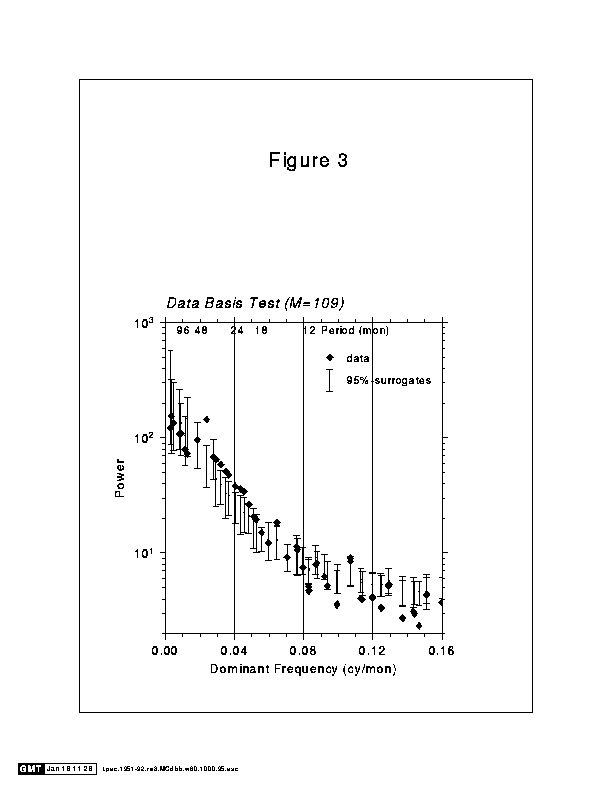
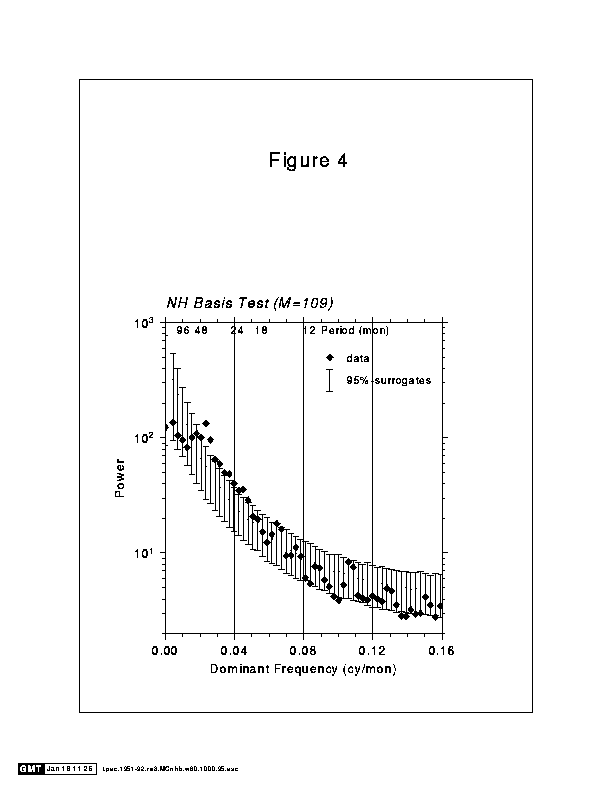
generalisation of the "Monte Carlo SSA" algorithm which allows an objective
test for the presence of oscillations at low signal-to-noise ratios in
multivariate data. The test issimilar to those used in standard regression,
examining directions in state-space to determine whether they contain more
varaince than would be expected if the noise null-hypothesis were valid.
We demonstrate the application of the test to the analysis of interannual
variability in tropical Pacific sea-surface temperatures.